{overleaf template gallery}Community articles — Recent

hw

Aditya Tikone's résumé. Created based on the Deedy CV template.

En este documento se explican qué son las matrices, los distintos tipos que hay, algunas operaciones que se hacen con ellas con ellas (y sus respectivas propiedades), además de enseñar cómo realizar estas operaciones en programación. Incluye el concepto de relación binaria, y se explica cómo se pueden representar éstas (si son homogéneas) mediante grafos. y los distintos tipos de éstos últimos. Se recalca la "matriz jacobiana", explicando su función escalar y vectorial

Een van de verbanden tussen magie en wiskunde is de anamorfosekunst. Een anamorfosekunstwerk is een vervormde tekening die haar ware aard pas laat zien wanneer ze vanuit een welbepaald standpunt bekeken wordt (de perspectivistische anamorfose) of wanneer ze weerspiegeld wordt in spiegel met een welbepaalde vorm (de spiegelanamorfose). In deze studie gaat het alleen over de perspectivistische anamorfosen. Wiskundig leggen ze een link met centrale projecties en met centraalperspectief.

A teaching plan

Christopher Amalraj's Curriculum Vitae

Vamos a demostrar el notable teorema que dice que, dadas dos matrices cuadradras \(A\) y \(B\) del mismo tamaño, si \(AB=I\), donde \(I\) es la matriz identidad del mismo tamaño que la matrices \(A\) y \(B\), entonces \(A\) es invertible y \(B^{-1}=A\). La prueba será directa y sólo usaremos el hecho de que si \(|A|\ne0\) entonces \(A\) es invertible. La pregunta es si puedes tú, estimado estudiante, ofrecer otra prueba de la que aquí se sugiere. Sirva además este texto como un ejemplo de escritura con LaTeX.

This project for a Calculus I class was adapted from a similar set of problems from the Calculus series by James Stewart.

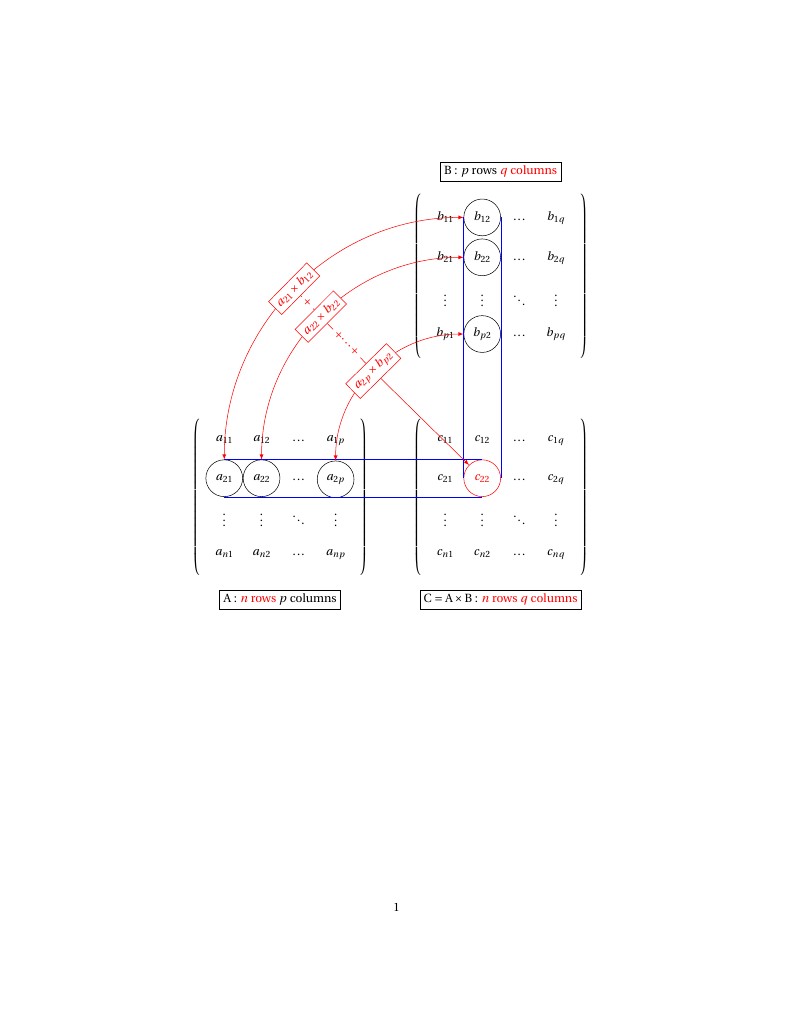
Graphical illustration explaining matrix multiplication