LaTeX templates and examples — Math
Recent
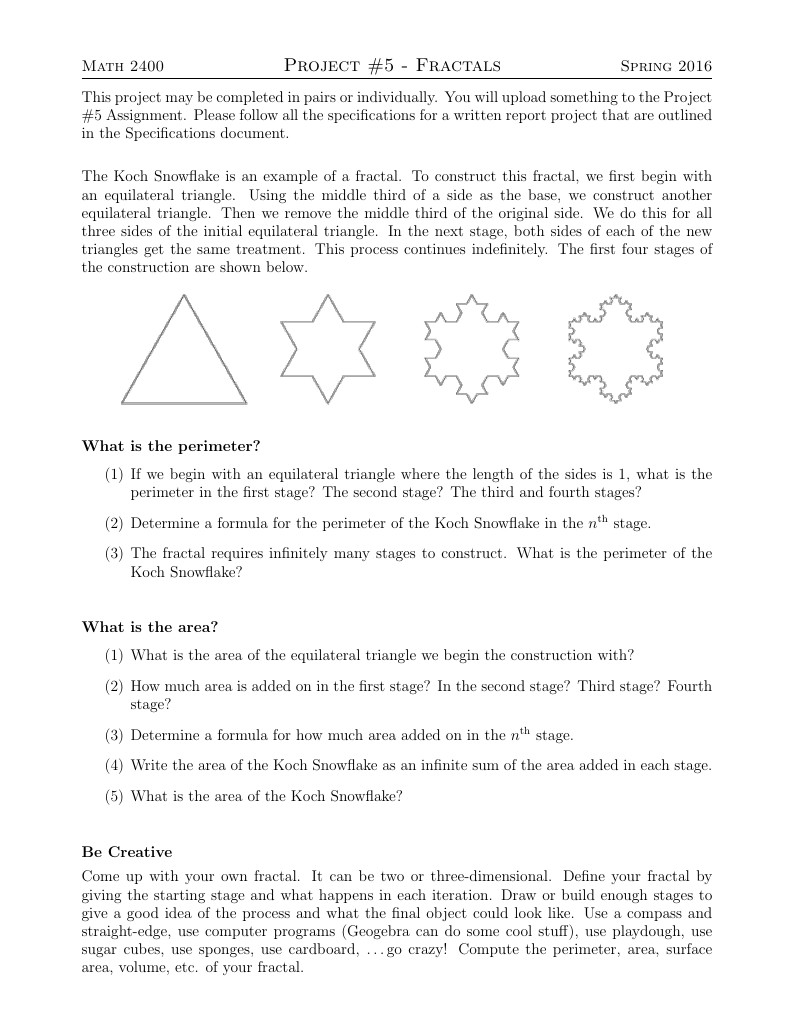
The fifth project for Spring 2017 Calculus 2 at Fitchburg State. This project covers fractals and geometric series.

This is the fourth project in Calculus 2 at Fitchburg State. Spring 2017.

A final assignment for measurement and error propagation in a sophomore physics lab course.
The second project for MATH 2400, Calculus II, at Fitchburg State. Estimating volume using definite integrals.
Example math quiz/exam in Vietnamese

“过去十年一涝,未来十年不涝的概率是?” Answer: 36.8% Though Poisson & Exponential are commonly used, few people understand why. This note exlpains why we use these two distribution.

Es posible diseñar modelos en donde la variable dependiente posea característica cualitativas, ese es el caso que analizaremos en el presente trabajo, enfocándonos únicamente en el modelo LOGIT que nos brinda ciertas ventajas en comparación a un modelo lineal de probabilidad, estimada por mínimos cuadrados ordinarios(MCO) para lo cual resaltaremos dichas diferencias. Los modelos de regresión con respuesta cualitativa son modelos de regresión en los cuales la variable dependiente puede ser de naturaleza cualitativa, mientras que las variables independientes pueden ser cualitativas o cuantitativas, o una mezcla de las dos; por ejemplo, si se está estudiando la relación entre ingresos y el pagar o no impuesto de renta, la respuesta o regresada solo puede tomar dos valores (si paga impuesto de renta o no paga dicho impuesto); otros ejemplos en que la regresada es cualitativa son si la familia posee o no vivienda propia, se aprueba o pierde un curso, padece determinada enfermedad o no la padece. La variable cualitativa en estos tipos de modelos no tiene que restringirse simplemente a respuestas de sí o no, la variable respuesta puede tomar más de dos valores, ser tricotómica o politómica, también se establecen modelos en lo que la variable dependiente es de carácter ordinal o de carácter nominal, en donde no hay preestablecido ningún tipo de orden. En este trabajo se analizara el modelo LOGIT en donde la variable dependiente es de carácter binario o dicotómico (sí o no). (Green 2001) Se trata pues de adoptar una formulación no lineal que obligue a que los valores estimados estén entre 0 y 1 ya que, la regresión con una variable binaria dependiente Y modeliza la probabilidad de que Y = 1. La regresión LOGIT utiliza una función de distribución logística, su función de distribución de probabilidad da lugar a probabilidades ente 0 y 1, y presenta un crecimiento no lineal (con mayores incrementos en la parte central).

The density of solid water, unlike most molecules, is less than that of its liquid form. Its precise value is of use in many applications. Freezing a spherical droplet of water and analyzing the changed shape from a sphere to a sphere with a slight peak in order to find the density of ice. We find the density of ice to be at 0.90 ± 1.66 · 106 g/mL. The precision of our measurement was limited by uncertainty in the angle measurements of the peak of the droplet.
\begin
Discover why over 20 million people worldwide trust Overleaf with their work.
