LaTeX templates and examples — Math

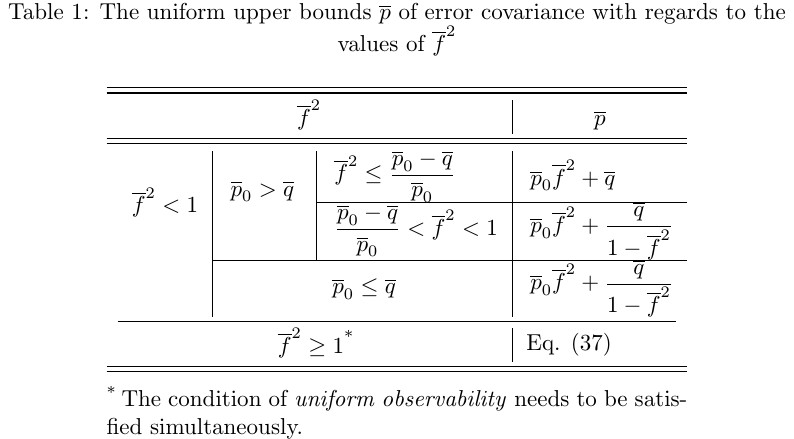
This is a table I used in the paper W. Li, G. Wei, D. Ding, Y. Liu and F. E. Alsaadi, "A New Look at Boundedness of Error Covariance of Kalman Filtering," in IEEE Transactions on Systems, Man, and Cybernetics: Systems, vol. 48, no. 2, pp. 309-314, Feb. 2018. I would like to share some LaTex codes for the table which might be helpful to some readers.

Modello di documento latex per la scrittura di soluzioni dei problemi (per la partecipazione agli stage delle olimpiadi di matematica).

In this project, students create a two-dimensional shape with nonuniform density, finds its center of mass, and hang it from a mobile. The various portion of the project address the differences and relationships between computing the center of mass of a discrete set of point masses and a lamina. Included here is also a sample solution to help students formulate their own well-written solutions. Also, in the LaTeX code are a few comments to address some of the basics of LaTeX and Overleaf.

hw

Initial template for students in Advanced Mathematical Perspectives 1 (AMP 1).

En este documento se explican qué son las matrices, los distintos tipos que hay, algunas operaciones que se hacen con ellas con ellas (y sus respectivas propiedades), además de enseñar cómo realizar estas operaciones en programación. Incluye el concepto de relación binaria, y se explica cómo se pueden representar éstas (si son homogéneas) mediante grafos. y los distintos tipos de éstos últimos. Se recalca la "matriz jacobiana", explicando su función escalar y vectorial

Les classes smfbook et smfart sont destinées à la composition en LaTeX des monographies et articles édités par la Société Mathématique de France.

The classes smfbook and smfart are intended to help the preparation in LaTeX of the monographs and articles to be published by the French Mathematical Society.

Vamos a demostrar el notable teorema que dice que, dadas dos matrices cuadradras \(A\) y \(B\) del mismo tamaño, si \(AB=I\), donde \(I\) es la matriz identidad del mismo tamaño que la matrices \(A\) y \(B\), entonces \(A\) es invertible y \(B^{-1}=A\). La prueba será directa y sólo usaremos el hecho de que si \(|A|\ne0\) entonces \(A\) es invertible. La pregunta es si puedes tú, estimado estudiante, ofrecer otra prueba de la que aquí se sugiere. Sirva además este texto como un ejemplo de escritura con LaTeX.